When I was in high school, a friend told me that she did not like mathematics because she found it too abstract. I was quite confused at the time and thought to myself: “But I love mathematics because it is abstract”. This made me quite curious: Is this disparity really about her disliking abstraction, and me loving it? Or did we actually mean different things when talking about abstraction? More concretely: What exactly do we mean when we qualify something as abstract? What exactly do we want when we wish something were more concrete? These questions are important, especially in mathematics education, because the very power of mathematics resides in abstraction. Now if anything abstract makes you cranky, I hope that this article will help you understand why some people can find excitement in abstraction. And if you live for abstraction, I hope that you will find some interesting new perspectives here.

Shocked

Over the past few months, I have been gathering definitions of “concrete” and “abstract”. For example, looking at only two papers about concreteness in math education [Fyfe 2014, Pouw 2014], I found that the word “concrete” was associated with: meaningful, familiar, well-understood, physical, grounded, pictural, perceptual, enactive, real-life, context-specific, and informal. In contrast, the word “abstract” is connected to: general, structural, portable, symbolic, vague, schematic. As we can see from these examples, some of these words do not exactly carry the same meaning. Saying that something is vague or unfamiliar is quite different from saying that something is structural and portable. Similarly, abstract art very much relates to our perceptual -- or so-called concrete -- abilities.

Artwork by artist Maja Malmcrona

In order to dig deeper, I asked several experts dealing with abstraction how they would define it. Let’s see how they perceive it, and why they are so intrigued by it.

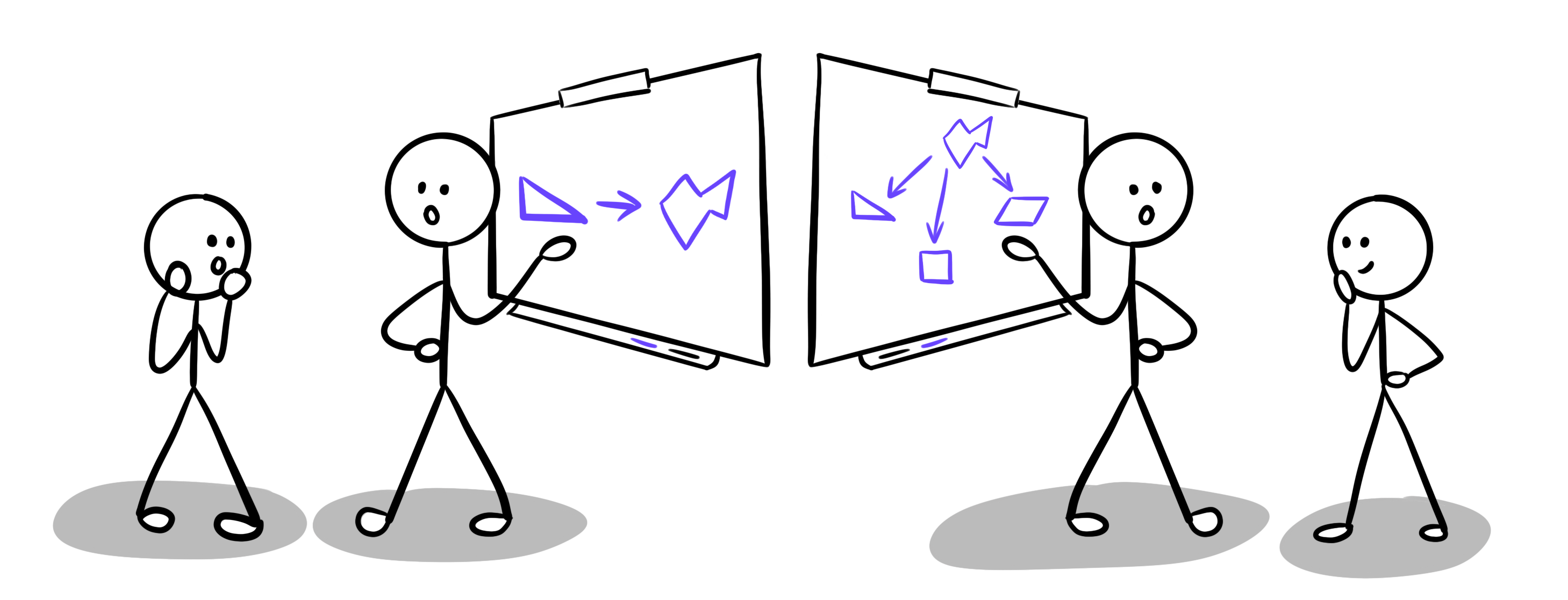
Because math is at the root of my quest, I started by asking Vera, a fellow mathematician, to see if we shared a similar perspective on abstraction. Vera answered my question like this: “Abstraction means ‘move to a higher level’. If you imagine objects to be hierarchically ordered according to their properties in a tree-shape (as for example species are ordered in biology), then abstraction means that you ‘zoom out’ to see the categories an object belongs to.”

Mathematician’s definition

According to Vera, abstraction is about looking at a certain situation from a higher perspective in the hierarchical tree of mathematical objects. And this is very powerful. For example, in the picture above, the tree shows that triangles and quadrilaterals are polygons, and that polygons are shapes. If we manage to prove a certain property at a high level, let’s say at the polygon level, this means that the property will be valid for all the shapes below that level. For example, we can prove that the sum of the internal angles of a polygon is equal to (number of sides - 2) * 180 degrees. This means that this formula will hold for all triangles, all squares, all dodecagons, and whatnot. If we did not look at things from this higher perspective, we would need to solve this problem for each shape one by one: a rather tedious endeavor. This defines abstraction as general.

And this leads us to our next definition, coming from a mathematician and philosopher, Zmaj: “Abstraction is developing the ability to, across different contexts, say ‘oh, this is the same’ and to actually use this sameness in a productive way.”

Philosophising mathematician’s definition

Once again, this definition ties with the idea of finding something similar, something common across different situations or different elements. However, this definition focuses more on the connections between these elements, rather than their category. Moreover, what is very interesting about this definition, is the explicit requirement of being able to use this understanding. It is not enough to notice that your wall is a big rectangle if you cannot apply what you know about rectangles to evaluate the amount of paint you need to cover the whole surface. This views abstraction as portable.

> the extra bit_

Computer scientists also spend a great deal of time playing with abstraction. Drew Hess summarizes abstraction as “the art of focusing only on the details that are relevant to a problem/concept/object/etc., and ‘forgetting’ all the others.” I think this is an important approach to consider, because it’s not so much about constructing an explicit abstraction. It’s about simplifying the problem by removing all the distracting elements. For example, back again to our wall-painting metaphor, you ought to wonder which features of the wall really matter when deciding on how much paint to buy: is it the height of the wall? its texture? its length? its thickness? its orientation with respect to the sun? the number of paintings hanging on it? Drew concludes by saying that “the goal is to make the solution/description/depiction as general as possible while still being effective in solving the problem or identifying the subject.”

Computer scientist’s definition

Bladyjoker states that abstraction is very important for programming. In particular, he explains that “programmers identify code similarities and differences which enables them to formulate a more general code form that is used to express the specific forms.” Concretely, programmers want to be able to reuse code as much as possible, in order to compress it and make it easier to maintain. Bladyjoker explains that some languages, such as Haskell, make it simple to see and extract these reusable components, while some others, such as Java, make it a bit more tedious. But Bladyjoker invites us to be careful when abstracting: if the abstraction gets too general, people might not be able to connect to it anymore, and end up not using the functionality.

Programmer’s definition

> the extra bit_over_

Now let’s move a bit further and look into linguistics, that is, the study of languages. KC, our undercover expert, explains that “Abstraction is the process of extracting from a diverse set of things some common and general pattern. Isn't it lovely that ‘abs-tract-ion’ and ‘ex-tract-ing’ share a root? Interestingly, in Arabic, ’tajriid’ (abstraction) comes from the root ‘jarada’, which means to remove, to strip.”

Linguist’s definition

This definition very much encompasses the idea of abstraction formulated by our first mathematician, Vera. We abstract by extracting the essential from a family of objects. On top of this, KC’s definition also insists on the aspect of removing superficial details to get to the essence of an object.

Now talking about essence, what do artists have to say about abstraction? Maja Malmcrona says that “To abstract, in art, is to unveil. It is an attempt to discover the essence of an experience by uncovering its most fundamental properties through the removal of superficial externals.” This relates very strongly to what we have seen when looking at science. But what does it mean for art? What is the essence of an experience? Maja explains that “Abstract art does not attempt to represent reality as we see it — but rather, it attempts to communicate reality as we feel it. It is not sourced from our external reality of objects and figures, but from our internal reality of concepts and emotions. It prefers sensations over visuals; universals over particulars.” And in this, we understand the process of abstract art. First, there is honesty and introspectivity, making you look directly at the changes that certain situations create within you. And then, there is the courage of putting it out there, and expressing it back to the world.

Artist’s definition

I feel there is some irony in portraying our internal models in the external world, through physical, and, one could say, concrete mediums. And, when we think about it, this irony can be found in all the fields we explored earlier in this article. Isn’t it interesting how mathematicians had to construct a whole new language of symbols in order to be able to communicate their understanding of the world? And in math like in art, the more you start understanding the language as well as your own internal representations, the more the abstract becomes relatable.

Abstract art and abstract math [1]

To conclude our quest, I asked a philosopher, anonymized as Ieva, for her thoughts on abstraction. Her answer was very thorough and interesting [2]. One comment that I found important to think about, is the fact that “’abstract’ can also be connoted negatively, to mean for something to be unduly removed from the more complex idea.” If there is no need for something to be abstracted from its context, if there is no need for us to reuse some underlying understanding anywhere else, why would we even bother with abstraction? Ieva also brought up the interesting idea that some things might be inherently abstract. Most of the definitions we saw so far focus on this idea of abstraction as starting from concreteness, and obtained by identifying common patterns, removing superficial details, or forming connections. But can we define abstraction without concrete instances? In this case, she says that “one solution is to create artificial ‘concreteness’, for example with educational technology that enables us to experience things that we cannot usually experience with our own senses. For example, we can create 3D animations of chemical processes that we could not see with our own eyes.” Here, we are moving the other way around. Similarly to what we saw with art, we are trying to concretize something abstract. Which leaves us with one question: “Is there anything lost in the process of 'de-abstraction'?”

Philosopher’s question

In this article, we asked experts from various domains for their definition of abstraction. In several fields, such as math and computer science, abstraction is presented as something general that can be reused in a productive way. This makes abstraction very useful because, with only one tool, we are now able to solve a myriad of problems. In order to build this productive abstraction, it is important to strip off the superficial details from concrete instances. This approach is mentioned by mathematicians, computer scientists, linguists, but also artists and philosophers. In all these fields, abstraction is about getting to the essential, the structural frame behind all the concrete objects and experiences.

All in all, we saw how experts agree on abstraction being portable, productive, and essential. But why do people sometimes use this word to describe something vague and unfamiliar? As we noticed, when presented naked, abstraction does not necessarily resemble anything we are likely to encounter in our everyday life. Even though you might be able to abstract a productive internal model from your concrete experiences, it is not always easy to express this understanding in the physical world. As we can see from mathematics and art, experts had to develop a specific language in order to be able to externalize this understanding and discuss it. If you do not speak this language and do not have the required support to learn it, you are very likely to miss the point and feel confused.

When using the word "abstract", people might indeed mean different things, or, rather, put their focus on different aspects of abstraction. As my friend said that math is too abstract for her, she was relating to the initial unfamiliarity of it all. And in return, when I looked at my own affection for abstraction, I was past the point of unfamiliarity and rather referring to its portability.

Teaching abstraction

Now if we come back to education, there are a few important things to understand. First, it might not always be reasonable to abstract right away. As Ieva mentioned, if abstraction is presented as only an alternative representation of something more concrete (and, in this case, more familiar), students will not have much incentive to remember and understand this foreign representation. And they might simply reject it. However, if abstraction is presented as a solution to several relatable problems, and is accompanied with the adapted tools to communicate about it, it suddenly becomes infinitely more valuable.

xoxo,

The Diverter

PS1: A big thank you to all the experts who contributed to the article and helped with proofreading!

PS2: The pretty art used in my not-so-pretty art was made by talented Maja Malmcrona and used with permission. In you're into art, philosophy, and life, go give her some love, because she's amazing! (website) (instagram) (podcast)

To go further:

The Ladder of Abstraction by Bret Victor.

You can also read this article:

Wagner, R., 2019. Mathematical Abstraction as Unstable Translation Between Concrete Presentations. Philosophy of Mathematics Education Journal, 35.

This excellent work describes mathematical abstraction as a translation between objects (or their representations) rather than the construction of some sort of ideal representation. The paper is grounded in the history of mathematics and mathematics education.

Notes:

[1] A Voronoi diagram in its natural habitat:

[2] The complete quote is: “’Abstract’ can be a characteristic of an idea or a thing. As a characteristic, to me, ‘abstract’ is the opposite of ‘concrete’, i.e. manifested in a context, or empirical. To describe something as abstract means to think of it as removed from specific occurrences, boiled down to the essence that allows us to recognise something as something. We abstract (as a verb) when we are trying to recognise something as something without experience. ’Abstract’ can also be connoted negatively, to mean for something to be unduly removed from the more complex idea. To me, it’s quite interesting to think of something - a thing or an idea - as inherently abstract, not as ‘abstracted’ from something. It seems that some things, for example particular concepts in Maths or Physics, but also in Art, we describe as inherently ‘abstract’. An abstract painting, for example, is not a reduction from a concrete and exact drawing of a ‘thing’; it is abstract and only abstract. In what way is this idea, i.e. of something being inherently abstract, related to the idea of ‘abstract’ as the opposite of ‘concrete’? I think this difference is quite important, especially if we think about learning and teaching. In the case of ‘abstract’ as the opposite of ‘concrete’, didactically we often assume that we should move from the concrete to the abstract: you first try something, and then you learn about the underlying ideas. But what if something is inherently abstract? One solution is to create artificial ‘concreteness’, for example with educational technology that makes experienceable things that we cannot experience with our own senses. For example, we can create 3D animations of chemical processes that we could not see with our own eyes. Another solution is to teach something (inherently) abstract as (inherently) abstract. Thinking about solution 1, I cannot help but wonder: What are we teaching? How can you teach something INHERENTLY abstract as concrete? Is there anything lost in the process of 'de-abstraction'?”

References:

[Fyfe 2014] Fyfe, E.R., McNeil, N.M., Son, J.Y. and Goldstone, R.L., 2014. Concreteness fading in mathematics and science instruction: A systematic review. Educational psychology review.

[Pouw 2014] Pouw, W.T., Van Gog, T. and Paas, F., 2014. An embedded and embodied cognition review of instructional manipulatives. Educational Psychology Review.